Stable component
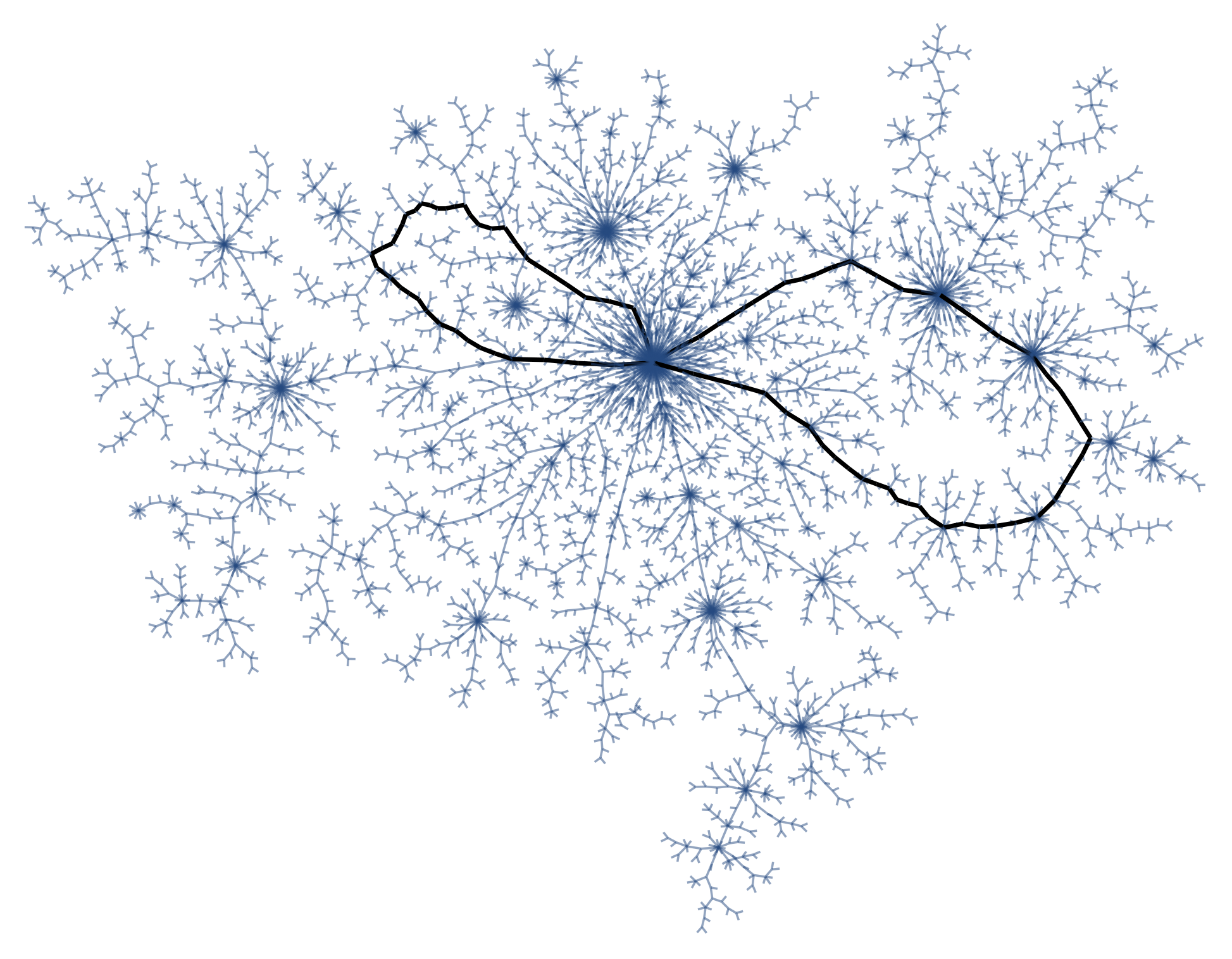
What is this?
This picture is an approximation of a realisation of the alpha-stable
component, for alpha=3/2. The alpha-stable component appears as the scaling
limit of a large connected component of a critical configuration model with
i.i.d degrees with a heavy tail, parametrised by a number alpha between 1
and 2.
Its local geometry corresponds to that of the alpha-stable tree, but it may
contain a finite number of cycles, which are drawn in black on the picture.
References
The scaling limit convergence of the critical configuration model with
i.i.d. degrees with a heavy-tailed distribution was proved in:
G. Conchon-Kerjan and C. Goldschmidt.
The stable graph: the metric space scaling limit of a critical random graph with i.i.d. power-law degrees. Preprint.
The distribution of the alpha-stable component was studied in:
C. Goldschmidt, B. Haas and D. Sénizergues.
Stable graphs: distributions and line-breaking construction.
Preprint.

