The Brownian map
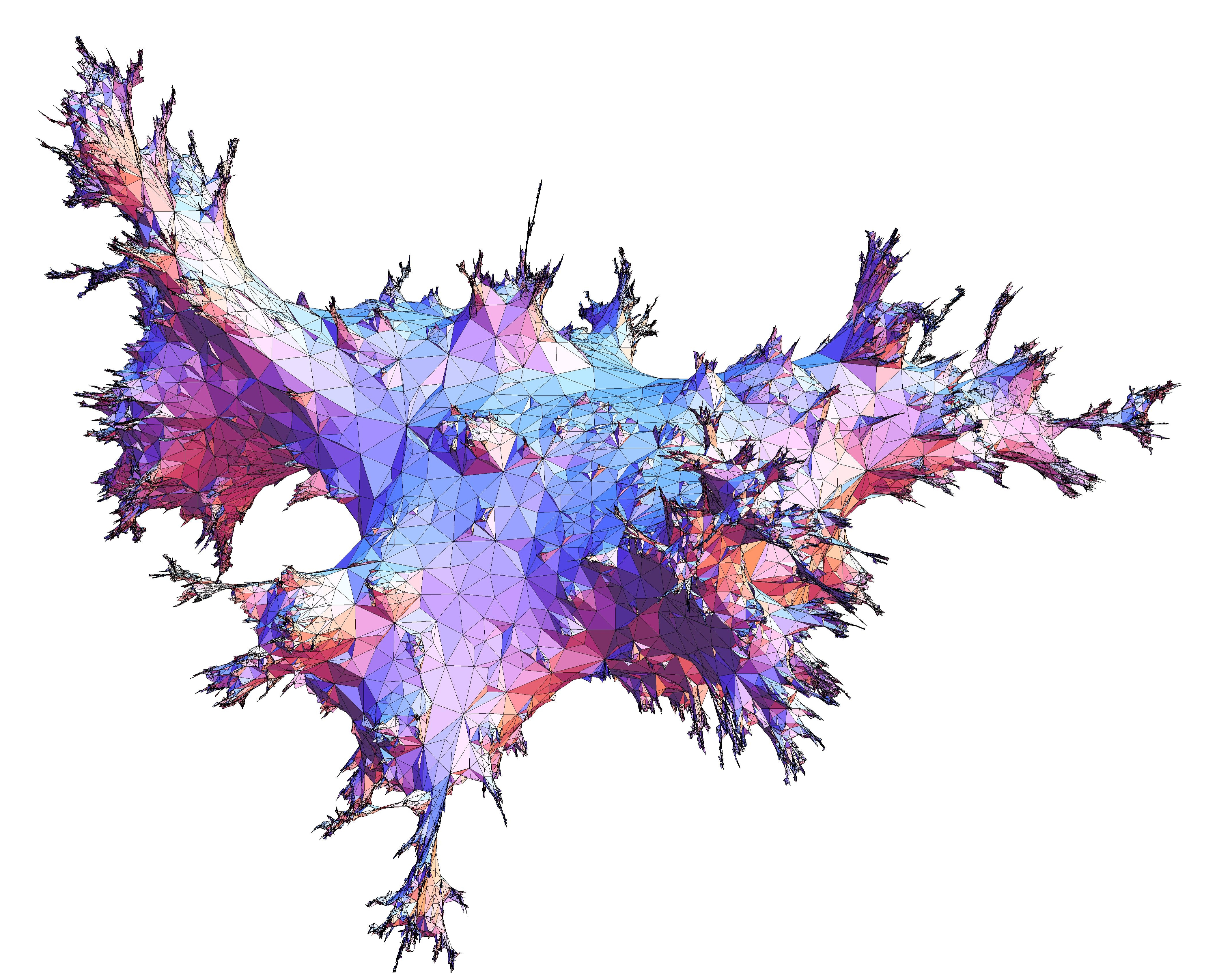
What is this?
This picture represents a random triangulation of the sphere, chosen
uniformly among all the triangulations with 30000 vertices. The sampling of
the random triangulation was done using edge-flips, and the embedding was
computed using the GraphPlot3D function of Mathematica.
An interesting feature of random planar maps is that the graph distances
between vertices in a uniform triangulation of size n are typically of
order n1/4. Moreover, after renormalizing distances by n1/4 a
uniform random triangulation converges in distribution to a random metric
space called the Brownian map. The picture above can be seen as an
approximation of the Brownian map.
Picture created by Thomas Budzinski.

